Mistakes involving incorrect cancellation are common but avoidable. Let's examine examples of several kinds of cancellation mistakes.

A common mistake is to cancel terms that are not common factors of either the numerator or denominator of a quotient of expressions.

Example 1: Simplify

Cancellation mistake:

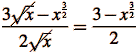
Click to see the mistake in red. Click to remove the red highlighting.

The √x can not be cancelled this way because it is not a common factor of the whole numerator.

Click to see a correct simplification. Click to hide the correct simplification.

In the correct simplification the first step is to write the numerator so that √x is a common factor; this means factoring √x from both terms of the numerator. Then √x can be cancelled.

There's a further technical note that can be important in some situations. Even when the quantity cancelled is (correctly) a common factor of both numerator and denominator, the cancellation is still often not universally valid. That's because there's an exception when the common factor takes the value zero. In Example 1 when x=0 the initial expression is undefined, since it reduces to the expression 0/0 (whereas the right side of the correct simplification computes to 3/2 when x=0). To be completely accurate, we should say that the simplification is correct whenever x ≠ 0. So: cancellation is not valid when the cancelled quantity is zero.

Taking care not to cancel zero can prevent another kind of cancellation mistake.

Example 2: Solve the equation

Cancellation mistake:

Click to see the mistake in red. Click to remove the red highlighting.

The mistake in cancelling x from both sides is precisely that the cancellation is not valid when x=0, and in fact x=0 is another solution of the equation. To avoid the mistake, first rearrange the equation to put all terms on one side (so it has the form "expression = 0") and then factor x. Try it yourself and then check the solution below.

Click to see a correct solution. Click to hide the correct solution.

It is usually safer to solve an equation like this by factoring since it eliminates the possibility of losing one or more solutions by invalid cancellation. The alternative is to carefully track exceptions (in this example, when x=0) whenever a cancellation is performed and separately check the special cases that arise from these exceptions. But remembering to track the exceptions is not as automatic as factoring, which is why I recommend the factoring method.

Another type of mistake occurs when function notation is not properly understood, or it is assumed that a function is linear.

Example 3: Can you simplify

?

Cancellation mistake:

Click to see the mistake in red. Click to remove the red highlighting.

What is wrong with this? Or, what justification would explain trying to cancel like this? The mistake may be based on one of two misunderstandings. One possibility is not understanding the function notation ln(3x). The expression ln(3x) means "take the natural logarithm of the quantity 3x". It does not mean multiply ln (whatever that would mean) by 3x. So 3 is not a factor of the numerator, and cannot be cancelled. A second possibility is mistakenly thinking that ln(3x) = 3 ln(x). This is the mistake of thinking that the natural logarithm function is linear. But only linear functions are linear! In fact, use of the correct logarithm rule allows the following "simplification", which does not result in any cancellation.

Click to see a correct "simplification". Click to hide the correct "simplification".

Eliminating cancellation mistakes is an important step in using algebra correctly. Take the time to cancel carefully!